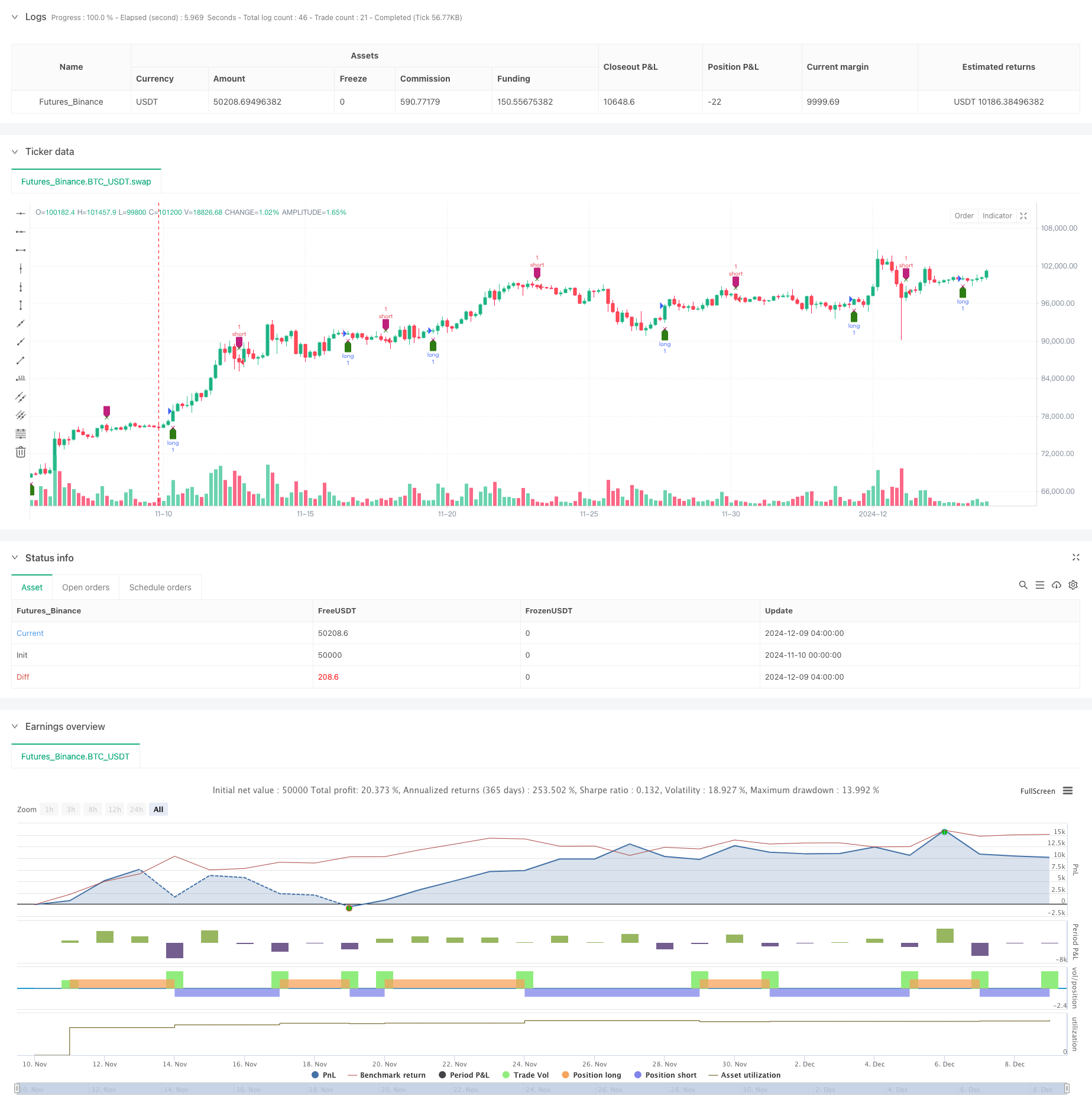
Die Strategie ist ein quantitatives Handelssystem, das auf einem dynamischen RSI-Oszillator basiert. Durch die Durchführung einer multivariaten Anpassung und einer Zeitreihenanalyse der RSI-Indikatoren wird die Veränderungsrate des RSI berechnet, um die Marktdynamik zu erfassen. Die Strategie verwendet fortgeschrittene mathematische Methoden wie die QR-Auflösung für die Signalverarbeitung und für die Handelsentscheidung in Verbindung mit einem linearen System.

Strategieprinzip

Der Delta-RSI-Obsolver steht im Mittelpunkt der Strategie und wird in folgenden Schritten umgesetzt:

1. Zuerst berechnen Sie den traditionellen RSI als Basis
2. RSI wird mit einer multiplen Anpassung glatter und geräuscharmer bearbeitet
3. Berechnen Sie die Zeitvariablen des RSI mit Delta-RSI, die die Veränderungsrate des RSI widerspiegeln
4. Vergleiche des Delta-RSI mit seinem Moving Average erzeugt Handelssignale
5. Bewertung und Filterung der Anpassungsklasse mittels der gleichmäßigen Quadratwurzel-Fehler (RMSE)

Die Handelssignale können auf drei Arten erzeugt werden:

• Null-Linien-Kreuzung: Delta-RSI ist positiver als negativ und null als negativ
• Signallinien kreuzen sich: Delta-RSI überschreitet/unterschreitet seinen Moving Average und macht Plus/Lose
• Richtungsänderung: Delta-RSI ist zu hoch, wenn der Negativbereich zu steigen beginnt, und zu niedrig, wenn der Positivbereich zu sinken beginnt

Strategische Vorteile

1. Die mathematische Basis ist solide. Die Signalverarbeitung erfolgt mittels fortgeschrittener mathematischer Methoden, wie z.B. der QR-Auflösung. Die theoretische Basis ist zuverlässig.
2. Signalglättung: Multi-Punkt-Einfügung filtert effektiv Marktlärm und verbessert die Signalqualität
3. Flexibilität: Verschiedene Signalgenerierungsmethoden und Parameteroptionen für verschiedene Marktumgebungen
4. Risikokontrolle: Ein RMSE-Filtermechanismus, der zuverlässige Signale auswählt
5. Hohe Rechenleistung: Die Matrix-Berechnungen werden durch optimierte Algorithmen effizienter ausgeführt

Strategisches Risiko

1. Parameter-sensibel: Mehrere Schlüsselparameter müssen sorgfältig angepasst werden, und die falsche Parameterwahl kann die Strategie-Performance erheblich beeinträchtigen
2. Verzögerung: Die glatte Verarbeitung des Signals führt zu einer gewissen Verzögerung und kann zu schnellen Verzögerungen führen.
3. Falsche Durchbrüche: Falsche Signale können in den turbulenten Märkten entstehen, was die Kosten erhöht.
4. Komplexe Berechnungen: Es sind mehr Matrix-Berechnungen erforderlich, und es kann zu Leistungsschwierigkeiten beim Hochfrequenz-Handel kommen.
5. Überpassung: Vorsicht bei der Optimierung von Parametern, um eine Überpassung der historischen Daten zu vermeiden

Richtung der Strategieoptimierung

1. Anpassungsparameter: RSI-Zyklen und Anpassungsstufen können entsprechend der Dynamik der Marktfluktuation angepasst werden
2. Mehrzeit-Zyklus: Signal mit mehr Zeit-Zyklen zu überprüfen
3. Fluktuationsratefilterung: Signalfilterung mit Fluktuationsrateindikatoren wie ATR
4. Marktklassifizierung: unterschiedliche Signalgenerationsregeln für verschiedene Marktzustände ((Trend/Schwankungen)
5. Stop-Loss-Optimierung: Hinzufügung von intelligenten Stop-Loss-Mechanismen, wie beispielsweise dynamische Stop-Loss-Basis auf unterstützenden Widerstandspunkten

Zusammenfassen

Es handelt sich um eine strukturierte, theoretisch solide und quantitativ ausgerichtete Handelsstrategie. Durch die Analyse der dynamischen Eigenschaften des RSI und die Signalverarbeitung in Verbindung mit modernen mathematischen Methoden ist es möglich, die Markttrends besser zu erfassen. Obwohl es einige Probleme mit der Parameterempfindlichkeit und der Rechenkomplexität gibt, hat die Strategie durch die vernünftige Auswahl und Optimierung von Parametern einen guten Anwendungswert.