Statistics 7 - The average number of cheaters

Statistics 7 - The average number of cheaters

Many people are confused about the mean, the median, the set, etc.

• There is a joke that is widely circulated, post it here, maybe it will help you understand it.

  M: Mr. Gizmodo has a small factory that produces super toys.

  M: The management consists of Mr. Gismo, his brother, six relatives. The staff consists of 5 clerks and 10 workers. The factory is running well and now needs a new worker.

  M: Now Mr. Gizmodo is meeting with Sam to talk about work.

  Gizmodo: We are well paid here. The average salary is 300 yuan a week. You get 75 yuan a week during your apprenticeship, but you can quickly process the money.

  M: After Sam worked for a few days, he asked to see the manager.

  Sam, you cheated me! I checked with other workers and none of them are paid more than $100 a week. How can the average wage be $300 a week?

  Gizmodo: Oh, Sam, don't get excited. The average salary is 300 yuan. I'll prove it to you.

  Gizmodo: This is my weekly salary. I get 2400 yuan, my brother gets 1000 yuan, my six relatives get 250 yuan each, five clerks get 200 yuan each, 10 workers get 100 yuan each.

  Sam: Yeah, yeah, yeah! You're right, the average salary is $300 a week.

  Gizmodo; I disagree! You really don't understand. I've listed a salary table and told you that the median salary is 200 yuan, but it's not the average salary, it's the median salary.

  Sam: What about 100 bucks a week?

  Gizmodo: The so-called "number" is the salary most people earn.

  Gizmodo: Big brother, your problem is that you don't know the difference between averages, medians and integers.

  Sam: Well, now I understand. I... I resign!

  This joke tells us the difference between the three parameters that describe the location of the data.

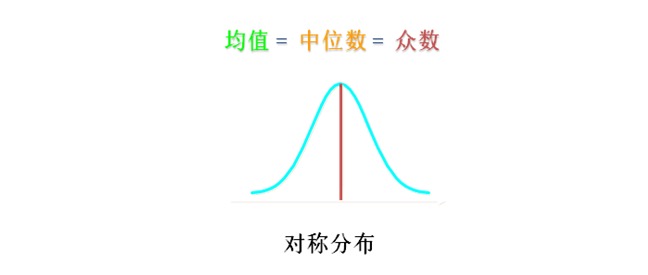
  In general, if the statistical distribution is symmetrical and the highest point is in the middle, then the mean, median, and integer are equal.

  

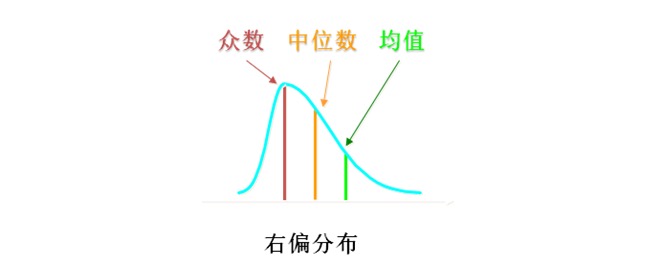
  If the statistical distribution is right-handed, i.e. mostly concentrated on the left, the right-hand side dragging a long tail, usually such as house prices, national income, etc. usually belongs to such a distribution, then in general the mean > median > number, when only looking at the mean may be one-sided, three parameters are needed to help you understand the data and the study object comprehensively.

  

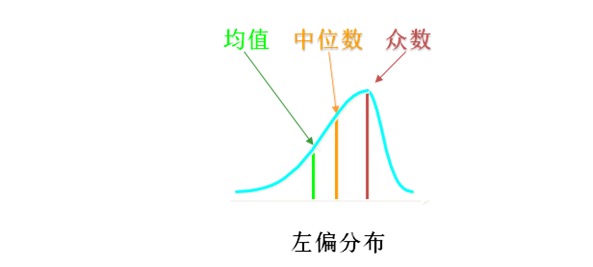
  The left-handed distribution has a right-handed distribution, and the mean is < median < number.

  

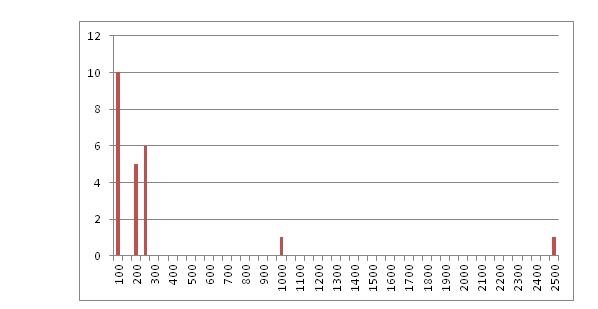
  In this example, the distribution looks like this:

  

Translated from the original Chinese by: