Gaussian se adapta a la estrategia de línea media

El autor:¿ Qué pasa?, Fecha: 2024-03-28 18:08:18
Las etiquetas:

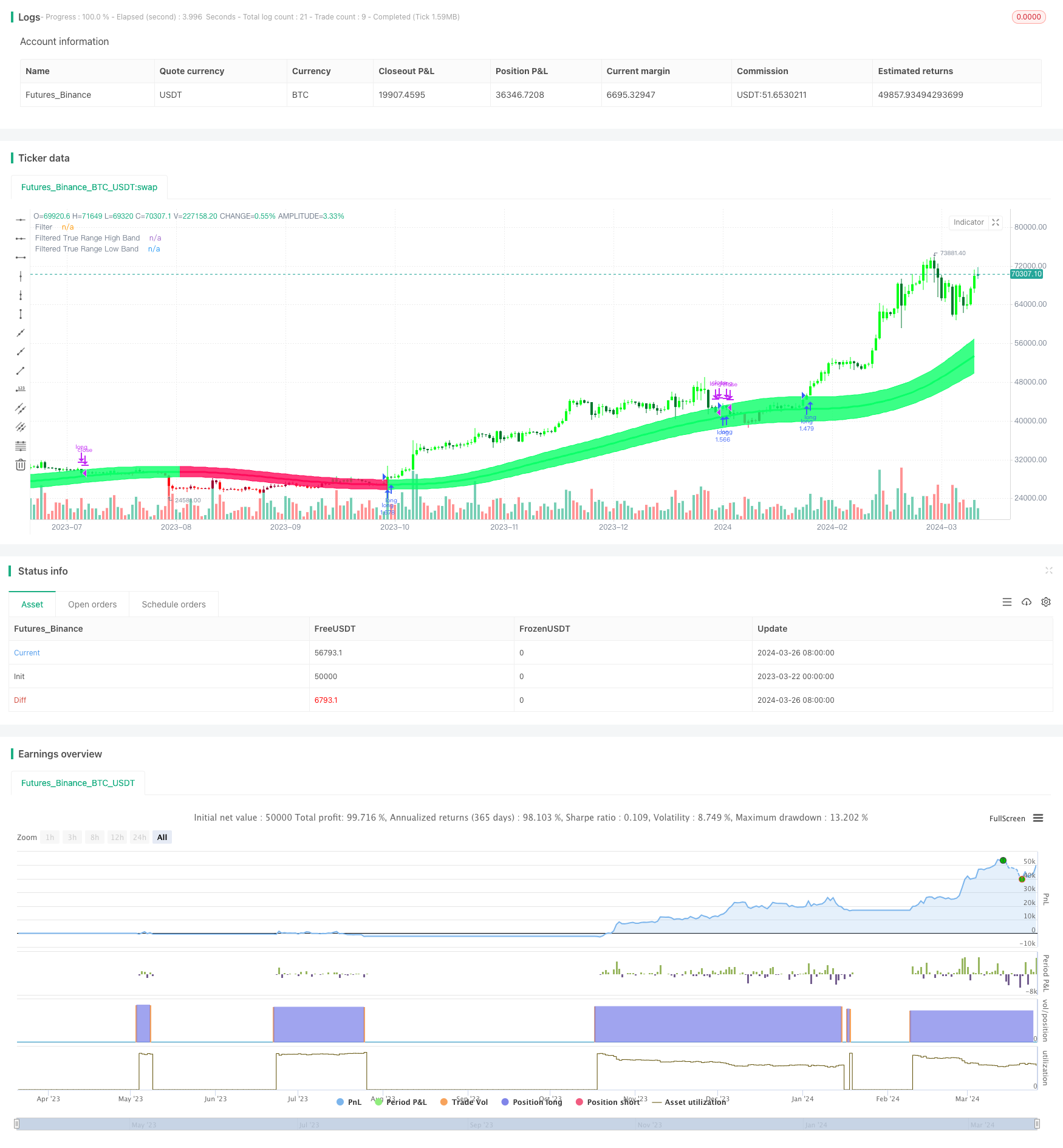
高斯通道自适应均线策略

Resumen

La estrategia del canal de Gauss es una estrategia de negociación cuantificada que utiliza la tecnología del canal de Gauss y el ajuste de parámetros de adaptación. La estrategia, basada en la teoría del canal de Gauss propuesta por John Ehlers, genera señales de negociación suaves y adaptables mediante el cálculo de múltiples medias móviles de índices sobre los datos de precios.

Principios estratégicos

Los principios de Gauss para adaptarse a la estrategia de línea media son los siguientes: 1. Calcula el valor de la onda de Gaussian en el precio. Se calculan los parámetros Beta y Alpha según el ciclo de muestra y el número de puntos de punto establecidos por el usuario, y luego se ejecutan ondas de Gaussian gradualmente en los datos de precios para obtener una secuencia de precios después de la limpieza. 2. Calcula el valor de Gauss de la amplitud de fluctuación real. El mismo tratamiento de Gauss de la amplitud de fluctuación real del precio se realiza para obtener una secuencia de amplitud de fluctuación suave. 3. Construir el canal de Gauss. Con el precio después de la onda de Gauss como canal central, el canal superior es el canal central, sumado al tamaño de la oscilación real por el multiplicador del valor establecido por el usuario, el canal inferior es el canal central y se reduce a este valor, formando un canal dinámico. Producción de señales de negociación. Producción de señales de compra cuando el precio rompe el canal hacia arriba; Producción de señales de venta cuando el precio rompe el canal hacia abajo. 5. Introducción de parámetros de intervalo de tiempo. El usuario puede configurar la hora en que la política comienza a funcionar y termina, durante la cual la política opera según las señales de negociación.

Análisis de ventajas

La estrategia de Gauss para adaptarse a la línea media tiene las siguientes ventajas: La estrategia utiliza parámetros de ajuste dinámico que se pueden adaptar a diferentes condiciones del mercado y variedades de transacciones, sin necesidad de frecuentes manipulaciones manuales. 2. Buen seguimiento de tendencias. Al construir un canal de precios, las estrategias pueden captar y seguir mejor las tendencias del mercado y evitar eficazmente las falsas señales en los mercados turbulentos. El uso de tecnología de alta frecuencia para el tratamiento de datos de precios de múltiples manejo suave, eliminando la mayor parte del ruido del mercado, lo que hace que las señales de negociación más confiables. 4. Alta flexibilidad. Los usuarios pueden ajustar los parámetros de la política, como el ciclo de muestreo, el número de puntos polares, el número de variantes, etc., según sea necesario, para optimizar el rendimiento de la política. 5. Gran utilidad. Se han introducido parámetros de intervalo de tiempo que permiten que las políticas se ejecuten en un período de tiempo especificado, lo que facilita la aplicación en el disco real y la investigación de retrospección.

Análisis de riesgos

A pesar de las ventajas de la estrategia de Gauss de adaptarse a la línea media, existen algunos riesgos: 1. El riesgo de la configuración de parámetros. La configuración inadecuada de parámetros puede causar el fracaso o el mal desempeño de la estrategia, por lo que se requiere una prueba y optimización repetidas en aplicaciones reales. 2. Riesgo de incidencias. En el caso de algunas incidencias importantes, las estrategias pueden no responder adecuadamente en el momento oportuno y causar pérdidas. 3. Riesgo de sobreajuste. Si los parámetros se ajustan demasiado a los datos históricos, la estrategia puede rendir mal en el futuro y necesitará tener en cuenta el rendimiento dentro y fuera de la muestra. 4. Riesgo de interés. La estrategia se aplica principalmente a los mercados tendenciales, y puede enfrentar un mayor riesgo de interés si se opera con frecuencia en mercados turbulentos.

Dirección de optimización

Las direcciones de optimización de Gauss para adaptarse a la estrategia de línea recta incluyen: 1. Optimización de parámetros dinámicos. Mejora la adaptabilidad mediante la introducción de tecnologías como el aprendizaje automático y el ajuste dinámico de parámetros estratégicos. 2. Fusión de múltiples factores. Combina otros indicadores o factores técnicos eficaces con el canal de Gauss para formar señales de negociación más sólidas. 3. Optimización de la gestión de posiciones. Incorporar reglas razonables de gestión de posiciones y gestión de fondos, control de retractos y riesgos, basadas en la estrategia. 4. Sinergia entre varias variedades. Extiende la estrategia a varias variedades de operaciones diferentes para dispersar el riesgo mediante la asignación de activos y el análisis de correlación.

Resumen

La estrategia de alta velocidad de adaptación a la línea recta es una estrategia de negociación cuantitativa basada en ondas de alta velocidad y parámetros de adaptación para generar señales de negociación suaves y confiables mediante la construcción dinámica de canales de precios. La estrategia tiene ventajas como una fuerte adaptabilidad, un buen seguimiento de tendencias, una gran suavidad, flexibilidad y utilidad, pero también enfrenta riesgos como configuración de parámetros, eventos repentinos, superajustes y alivios. En el futuro, la estrategia puede ser mejorada y mejorada en términos de optimización de parámetros dinámicos, fusión de factores, diversificación de la gestión de posiciones, sinergia de múltiples variedades.

/*backtest
start: 2023-03-22 00:00:00
end: 2024-03-27 00:00:00
period: 1d
basePeriod: 1h
exchanges: [{"eid":"Futures_Binance","currency":"BTC_USDT"}]
*/

//@version=4
strategy(title="Gaussian Channel Strategy v1.0", overlay=true, calc_on_every_tick=false, initial_capital=10000, default_qty_type=strategy.percent_of_equity, default_qty_value=100, commission_type=strategy.commission.percent, commission_value=0.1)

// Date condition inputs
startDate = input(title="Date Start", type=input.time, defval=timestamp("1 Jan 2018 00:00 +0000"), group="Dates")
endDate = input(title="Date End", type=input.time, defval=timestamp("31 Dec 2060 23:59 +0000"), group="Dates")
timeCondition = true

// This study is an experiment utilizing the Ehlers Gaussian Filter technique combined with lag reduction techniques and true range to analyze trend activity.
// Gaussian filters, as Ehlers explains it, are simply exponential moving averages applied multiple times.
// First, beta and alpha are calculated based on the sampling period and number of poles specified. The maximum number of poles available in this script is 9.
// Next, the data being analyzed is given a truncation option for reduced lag, which can be enabled with "Reduced Lag Mode".
// Then the alpha and source values are used to calculate the filter and filtered true range of the dataset.
// Filtered true range with a specified multiplier is then added to and subtracted from the filter, generating a channel.
// Lastly, a one pole filter with a N pole alpha is averaged with the filter to generate a faster filter, which can be enabled with "Fast Response Mode". 

//Custom bar colors are included.

//Note: Both the sampling period and number of poles directly affect how much lag the indicator has, and how smooth the output is.
//      Larger inputs will result in smoother outputs with increased lag, and smaller inputs will have noisier outputs with reduced lag.
//      For the best results, I recommend not setting the sampling period any lower than the number of poles + 1. Going lower truncates the equation.

//-----------------------------------------------------------------------------------------------------------------------------------------------------------------
//Updates:
// Huge shoutout to @e2e4mfck for taking the time to improve the calculation method!
// -> migrated to v4
// -> pi is now calculated using trig identities rather than being explicitly defined.
// -> The filter calculations are now organized into functions rather than being individually defined.
// -> Revamped color scheme.

//-----------------------------------------------------------------------------------------------------------------------------------------------------------------
//Functions - courtesy of @e2e4mfck
//-----------------------------------------------------------------------------------------------------------------------------------------------------------------
 
//Filter function 
f_filt9x (_a, _s, _i) => 
    int _m2 = 0, int _m3 = 0, int _m4 = 0, int _m5 = 0, int _m6 = 0, 
    int _m7 = 0, int _m8 = 0, int _m9 = 0, float _f = .0, _x = (1 - _a)
    // Weights. 
    // Initial weight _m1 is a pole number and equal to _i
    _m2 := _i == 9 ? 36  : _i == 8 ? 28 : _i == 7 ? 21 : _i == 6 ? 15 : _i == 5 ? 10 : _i == 4 ? 6 : _i == 3 ? 3 : _i == 2 ? 1 : 0
    _m3 := _i == 9 ? 84  : _i == 8 ? 56 : _i == 7 ? 35 : _i == 6 ? 20 : _i == 5 ? 10 : _i == 4 ? 4 : _i == 3 ? 1 : 0
    _m4 := _i == 9 ? 126 : _i == 8 ? 70 : _i == 7 ? 35 : _i == 6 ? 15 : _i == 5 ? 5  : _i == 4 ? 1 : 0
    _m5 := _i == 9 ? 126 : _i == 8 ? 56 : _i == 7 ? 21 : _i == 6 ? 6  : _i == 5 ? 1  : 0 
    _m6 := _i == 9 ? 84  : _i == 8 ? 28 : _i == 7 ? 7  : _i == 6 ? 1  : 0 
    _m7 := _i == 9 ? 36  : _i == 8 ? 8  : _i == 7 ? 1  : 0 
    _m8 := _i == 9 ? 9   : _i == 8 ? 1  : 0 
    _m9 := _i == 9 ? 1   : 0
    // filter
    _f :=   pow(_a, _i) * nz(_s) + 
      _i  *     _x      * nz(_f[1])      - (_i >= 2 ? 
      _m2 * pow(_x, 2)  * nz(_f[2]) : 0) + (_i >= 3 ? 
      _m3 * pow(_x, 3)  * nz(_f[3]) : 0) - (_i >= 4 ? 
      _m4 * pow(_x, 4)  * nz(_f[4]) : 0) + (_i >= 5 ? 
      _m5 * pow(_x, 5)  * nz(_f[5]) : 0) - (_i >= 6 ? 
      _m6 * pow(_x, 6)  * nz(_f[6]) : 0) + (_i >= 7 ? 
      _m7 * pow(_x, 7)  * nz(_f[7]) : 0) - (_i >= 8 ? 
      _m8 * pow(_x, 8)  * nz(_f[8]) : 0) + (_i == 9 ? 
      _m9 * pow(_x, 9)  * nz(_f[9]) : 0)

//9 var declaration fun
f_pole (_a, _s, _i) =>
    _f1 =            f_filt9x(_a, _s, 1),      _f2 = (_i >= 2 ? f_filt9x(_a, _s, 2) : 0), _f3 = (_i >= 3 ? f_filt9x(_a, _s, 3) : 0)
    _f4 = (_i >= 4 ? f_filt9x(_a, _s, 4) : 0), _f5 = (_i >= 5 ? f_filt9x(_a, _s, 5) : 0), _f6 = (_i >= 6 ? f_filt9x(_a, _s, 6) : 0)
    _f7 = (_i >= 2 ? f_filt9x(_a, _s, 7) : 0), _f8 = (_i >= 8 ? f_filt9x(_a, _s, 8) : 0), _f9 = (_i == 9 ? f_filt9x(_a, _s, 9) : 0)
    _fn = _i == 1 ? _f1 : _i == 2 ? _f2 : _i == 3 ? _f3 :
      _i == 4     ? _f4 : _i == 5 ? _f5 : _i == 6 ? _f6 :
      _i == 7     ? _f7 : _i == 8 ? _f8 : _i == 9 ? _f9 : na
    [_fn, _f1]

//-----------------------------------------------------------------------------------------------------------------------------------------------------------------
//Inputs
//-----------------------------------------------------------------------------------------------------------------------------------------------------------------

//Source
src = input(defval=hlc3, title="Source")

//Poles
int N = input(defval=4, title="Poles", minval=1, maxval=9)

//Period
int per = input(defval=144, title="Sampling Period", minval=2)

//True Range Multiplier
float mult = input(defval=1.414, title="Filtered True Range Multiplier", minval=0)

//Lag Reduction
bool modeLag  = input(defval=false, title="Reduced Lag Mode")
bool modeFast = input(defval=false, title="Fast Response Mode")

//-----------------------------------------------------------------------------------------------------------------------------------------------------------------
//Definitions
//-----------------------------------------------------------------------------------------------------------------------------------------------------------------

//Beta and Alpha Components
beta  = (1 - cos(4*asin(1)/per)) / (pow(1.414, 2/N) - 1)
alpha = - beta + sqrt(pow(beta, 2) + 2*beta)

//Lag
lag = (per - 1)/(2*N)

//Data
srcdata = modeLag ? src + (src - src[lag]) : src
trdata  = modeLag ? tr(true) + (tr(true) - tr(true)[lag]) : tr(true)

//Filtered Values
[filtn, filt1]     = f_pole(alpha, srcdata, N)
[filtntr, filt1tr] = f_pole(alpha, trdata,  N)

//Lag Reduction
filt   = modeFast ? (filtn + filt1)/2 : filtn
filttr = modeFast ? (filtntr + filt1tr)/2 : filtntr

//Bands
hband = filt + filttr*mult
lband = filt - filttr*mult

// Colors
color1   = #0aff68
color2   = #00752d
color3   = #ff0a5a
color4   = #990032
fcolor   = filt > filt[1] ? #0aff68 : filt < filt[1] ? #ff0a5a : #cccccc
barcolor = (src > src[1]) and (src > filt) and (src < hband) ? #0aff68 : (src > src[1]) and (src >= hband) ? #0aff1b : (src <= src[1]) and (src > filt) ? #00752d : 
           (src < src[1]) and (src < filt) and (src > lband) ? #ff0a5a : (src < src[1]) and (src <= lband) ? #ff0a11 : (src >= src[1]) and (src < filt) ? #990032 : #cccccc

//-----------------------------------------------------------------------------------------------------------------------------------------------------------------
//Outputs
//-----------------------------------------------------------------------------------------------------------------------------------------------------------------

//Filter Plot
filtplot = plot(filt, title="Filter", color=fcolor, linewidth=3)

//Band Plots
hbandplot = plot(hband, title="Filtered True Range High Band", color=fcolor)
lbandplot = plot(lband, title="Filtered True Range Low Band", color=fcolor)

//Channel Fill
fill(hbandplot, lbandplot, title="Channel Fill", color=fcolor, transp=80)

//Bar Color
barcolor(barcolor)


longCondition = crossover(close, hband) and timeCondition
closeAllCondition = crossunder(close, hband) and timeCondition

if longCondition
    strategy.entry("long", strategy.long)

if closeAllCondition
    strategy.close("long")

Más contenido

2019 - FMZ - Cuantificación de los inventores